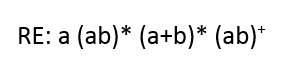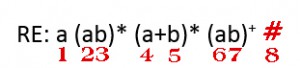Irving's Blog

Soal:

Buat RE -> DFA dengan 2 cara:

• Menggunakan cara tree
• Cara ε – NFA

Setelah itu lakukan DFA minimized.

Constraint:

• State dari DFA minimal 5 state maksimal 8 state.
• Final state minimal 2 state dan maksimal 3 state

Jawaban:

1. Menggunakan cara tree

Tentukan index terlebih dahulu:

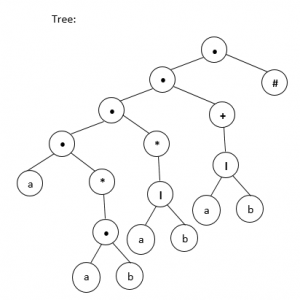
Buat tree-nya:

Kemudian, tentukan followpost masing – masing index:

• Follow post 1 = 2, 4, 5, 6
• Follow post 2 = 3
• Follow post 3 = 2, 4, 5, 6
• Follow post 4 = 4, 5, 6
• Follow post 5 = 4, 5, 6
• Follow post 6 = 7
• Follow post 7 = 6, 8
• Follow post 8 = –

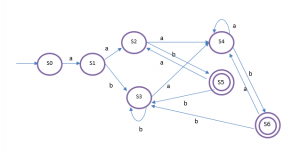
Berdasarkan followpost di atas, tentukan state – statenya:

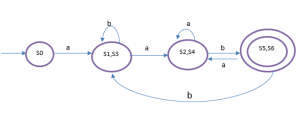
Hasil DFA:

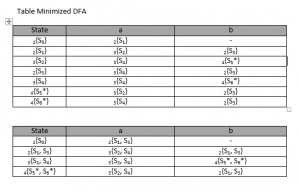
DFA di atas masih dapat kita minimalisasi dengan melakukan grouping, sebagai berikut:

Hasil DFA setelah diminimized:

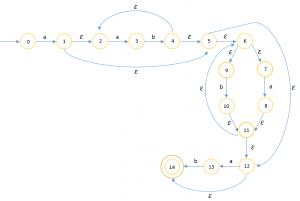
2. Menggunakan cara ε – NFA

Pertama, buat ε – NFAnya:

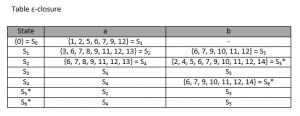
Kemudian cari ε-closure movenya:

• ε-closure (0) = 0 → {S₀}
• ε-closure (move(S₀, a)) = ε-closure {1} = {1, 2, 5, 6, 7, 9, 12} → {S₁}
• ε-closure (move(S₀, b)) = {}
• ε-closure (move(S₁, a)) = ε-closure {3, 8, 13} = {3, 6, 7, 8, 9, 11, 12, 13} → {S₂}
• ε-closure (move(S₁, b)) = ε-closure {10} = {6, 7, 9, 10, 11, 12} → {S₃}
• ε-closure (move(S₂, a)) = ε-closure {8, 13} = {6, 7, 8, 9, 11, 12, 13} → {S₄}
• ε-closure (move(S₂, b)) = ε-closure {4, 10, 14} = {2, 4, 5, 6, 7, 9, 10, 11, 12, 14} → {S₅*}
• ε-closure (move(S₃, a)) = ε-closure {8, 13} = {S₄}
• ε-closure (move(S₃, b)) = ε-closure {10} ={S₃}
• ε-closure (move(S₄, a)) = ε-closure {8, 13} = {S₄}
• ε-closure (move(S₄, b)) = ε-closure {10, 14} = {6, 7, 9, 10, 11, 12, 14} → {S₆*}
• ε-closure (move(S₅, a)) = ε-closure {3, 8, 13} = {S₂}
• ε-closure (move(S₅, b)) = ε-closure {10} = {S₃}
• ε-closure (move(S₆, a)) = ε-closure {8, 13} = {S₄}
• ε-closure (move(S₆, b)) = ε-closure {10} = {S₃}

Dari langkah di atas dapat ditentukan jalur masing – masing statenya:

Hasil DFA:

http://binus.ac.id